Projection (mathematics)

Generally speaking, in mathematics, a projection is a mapping of a set (or of a mathematical structure) which is idempotent, which means that a projection is equal to its composition with itself. A projection may also refer to a mapping which has a left inverse. Both notions are strongly related, as follows. Let p be an idempotent map from a set E into itself (thus p∘p = Id_E) and F = p(E) be the image of p. If we denote by π the map p viewed as a map from E onto F and by i the injection of F into E, then we have i∘π = Id_F. Conversely, i∘π = Id_F implies that π∘i is idempotenT.

Originally, the notion of projection was introduced in Euclidean geometry to denotes the projection of the Euclidean space of dimension three onto a plane in it. The two main projections of this kind are

The projection from a point onto a plane or central projection: If C is the point, called center of projection, the projection of a point P different of C is the intersection with the plane of the line CP. The point C and the points P such that the line CP is parallel to the plane do not have any image by the projection.
The projection onto a plane parallel to a direction D: The image of a point P is the intersection with the plane of the line parallel to D passing through P.

Various other projections, called projection maps have been defined for the need of cartography. The 3D projections are also at the basis of the theory of perspective.

The need of unifying the two kinds of projections and of defining the image by a central projection of any point different of the center of projection are at the origin of projective geometry.

The original notion of projection has been extended or generalized to various mathematical situations, frequently, but not always, related to geometry, for example:

In set theory:
- An operation typified by the j ^th projection map, written proj_j, that takes an element x = (x₁, ..., x_j, ..., x_k) of the cartesian product X₁ × … × X_j × … × X_k to the value proj_j(x) = x_j. This map is always surjective.
- A mapping that takes an element to its equivalence class under a given equivalence relation is known as the canonical projection.
- The evaluation map sends a function f to the value f(x) for a fixed x. The space of functions Y^X can be identified with the cartesian product 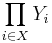$\prod_{i\in X}Y_i$ , and the evaluation map is a projection map from the cartesian product.
In category theory, the above notion of cartesian product of sets can be generalized to arbitrary categories. The product of some objects has a canonical projection morphism to each factor. This projection will take many forms in different categories. The projection from the Cartesian product of sets, the product topology of topological spaces (which is always surjective and open), or from the direct product of groups, etc. Although these morphisms are often epimorphisms and even surjective, they do not have to be.
In linear algebra, a linear transformation that remains unchanged if applied twice (p(u) = p(p(u))), in other words, an idempotent operator. For example, the mapping that takes a point (x, y, z) in three dimensions to the point (x, y, 0) in the plane is a projection. This type of projection naturally generalizes to any number of dimensions n for the source and k ≤ n for the target of the mapping. See orthogonal projection, projection (linear algebra). In the case of orthogonal projections, the space admits a decomposition as a product, and the projection operator is a projection in that sense as well.
In differential topology, any fiber bundle includes a projection map as part of its definition. Locally at least this map looks like a projection map in the sense of the product topology, and is therefore open and surjective.
In topology, a retract is a continuous map r: X → X which restricts to the identity map on its image. This satisfies a similar idempotency condition r² = r and can be considered a generalization of the projection map. A retract which is homotopic to the identity is known as a deformation retract. This term is also used in category theory to refer to any split epimorphism.
The scalar projection (or resolute) of one vector onto another.